ISSN 0798 1015

Vol. 39 (# 21) Year 2018. Page 27

The importance of mathematical sciences in the development of future specialists’ potential in socioeconomic researching

La importancia de las ciencias matemáticas en el desarrollo del potencial de futuros especialistas en investigación socioeconómica

Marina G. SERGEEVA 1; Ivan A. PUGACHEV 2; Marina B. BUDILTSEVA 3; Irina Yu. VARLAMOVA 4; Natalya S. NOVIKOVA 5; Natalia V. GENINA 6

Received: 19/01/2018 • Approved: 01/02/2018

Contents

1. Introduction

2. Methodology of Research

3. Research results

4. Discussion

5. Conclusion

References

1. Introduction

Recent trends of complexity and modernization in modern society have enhanced strategies to facilitate the training of scientists capable to shape a social and economic landscape, analyze social requirements, articulate more efficient novel strategies to meet the challenges in production areas and anticipate different changes in a society. As a result, training specialists in socio-economic areas (later SEA) turns out to be one of the most relevant criteria. (Belenchuk 2016).

Academic paper comparative research has shown that the extent of scientific investigation of training professional researchers in humanitarian areas are the highest in pedagogics. Less attention has been paid to this concept in education of IT specialists and sociologists. Therefore, it is urgent to develop the principles and generate the methods of training specialists in different fields: managers, executives, sociologists and others.

Based on the analysis of the research data carried out according to the professional training methods (Sukhodimtseva 2017), we have distinguished five most promising complementary methodological approaches for SEA specialists training, which fall into systematic, cultural, competence, contextual, activity domains.

A systematic approach addresses research readiness as a system of interrelated and interdependent components. The advantages of systematic approach give a teacher insight to succeed in educating different aspects of trainees.

A cultural approach elaborates the impact of educational environment adopted to students’ different cultural background. It facilitates human potential, encourages self-improvement and position in profession.

A competence approach empowers individuals with the knowledge they will require to carry out scientific research and integrate relevant knowledge quality improvement into daily work. E. Nikitina treats this approach as fundamental. (Nikitina 2014b)

A contextual approach plays a key role in researcher’s education. It facilitates achievements in professional skills and helps to get knowledge through modelling professional environment (both domain-specific component and social one) and through definite deductive tools. (Nikitina 2016).

 An activity approach facilitates analyses and research process modeling in terms of activity theory. It predetermines that a student is the subject of both educational activity and pedagogical interaction. (Korzhuyev 2009)

These approaches form the basis for the suggested model to develop future SEA specialist readiness for research within the framework of the higher mathematical education.

2. Methodology of Research

Nowadays mathematics ranks high in socio-economics, and mastery of conceptual notion, methods and models within this subject defines its relevancy for managers, marketing specialists, sociologists. Thus, it is necessary to empower students of particular specialties with core competency in mathematical database objects and notions, facilitate crucial knowledge-based mode to fulfill practical professional tasks, familiarize them with main sources of information about different mathematical methods and the way to use them in economics and sociology research issues. It will inherently serve to practice deep insight into three basic things: means of computation, a research method and a universal language. It will drive SAE students to realize the importance of mathematics in forming and developing a research component in professional readiness. (Sukhodimtseva 2016).

N. Prosolupova (2011) and M. Sergeeva (2015) are highly experienced in teaching mathematics to art students. These students are often reluctant in studying mathematics and treat it like an optional in the pursuit of professional consistency, reasoning for different aptitudes and interests. In this sense, these results trace back to poor performance in the course of mathematics at school, lack of skills in autonomous learning, an attempt to avoid the wrong alternative in choosing a profession (most of them do not find mathematics to be a direct object of study for their future occupation).

Traditionally mathematics for art students falls into two teaching approaches. The first one provides this subject as general not specialized and aims at raising a standard educational level for art students. The adherents of the second approach believe that mathematics teaching should facilitate the skills and knowledge for students’ maturity in profession. The authors of this article are supporters of the latter approach. Profession-oriented teaching will definitely bring about the required change in motivation of learning and education in general for maximum benefits and will affect a prosperous profession. Analyses of the course in mathematics, some means and assignments in profession-oriented teaching, which modern scientists have analyzed, prove the necessity of supplement research component in the present course. Its particular characteristics are the following: - mathematical simulation is used in teaching for trainees to be acquainted with mathematical methods in researching to shape their professional orientation, to develop their own reflexive- research opinions. Stage-by-stage formation of professional competence, which is used to vary the comprehension level of difficulties, is certain to be the best choice that works for addressing art students. In other words, it creates environments to implement two most important principles in pedagogics: continuity and consequentiality. The mathematical simulation courses, obviously, should be included in the curriculum for the first-year SEA students. It demands to include some supplementary tasks as well as some creative professionally- oriented situations to empower the educational process. This simulation-based activity promotes knowledge consolidation and makes them relevant to scientific research, boosts basic skills in hypothesizing, model studying, conceptual foresight, problem framing and making a conclusion. (Nikitina 2014a)

Therefore, mathematical simulation is the tool SEA students can use to study processes in social and economic areas as well as the method, first, (1) to develop research reasoning and reflexive skills; second, (2) students’ involvement in mastering their knowledge in these fields. The implementation of the resources mentioned above is efficiency-enhancing in the following functions of the higher mathematical education:

Educational: thecreation of models demands the classification of the available target-of-research data results and it significantly effects intellectual work (in terms of analysis, synthesis, generalization, comparison, classification, similarity method and other cogitative operations);

Self-directed learning: mathematical simulation structures thinking processes (both at common and professional levels) empower students’ cognitive abilities;

Prognostic: it demands hypothesis formulation, solution path analyses. Students are likely to speculate possible research outcomes, anticipate possible consequences of some difficulties through mathematical principles in this model;

Motivational: the analysis of definite situations promotes students’ proactive training, i.e. it develops skills and competences integrated into their future profession;

Reflexive: mathematical simulation correlates to self-potential analysis, task-reasoning generation, reflective and cognitive activity.

3. Research results

The analysis of authors’ proceedings in which they have scrutinized the research components in students’ training (Prosolupova 2011; Sergeeva 2015) enables to explicate a working definition of the notion “SEA students’ research readiness”. This notion is defined as a person-oriented systematic education which ensures effective professional activity within the frame of occupation choice, and which falls into five different domains: motivational, cognitive, active-practical, reflexive, peronal-creative components (Sukhodimtseva 2014).

The first component is a system of predominant motives (social, cognitive, professional) a student has, and which determines his or her attitude towards scientific research and its structural elements.

A cognitive component, essential for SEA specialists, embodies theoretical information complex (thesaurus included), to meet the challenge in research-based tasks and to elaborate on its basis students’ reasoning in the professional areas (in terms of the person’s skills, knowledge and aptitude). Cognitive component includes general-theoretical, technological and special domains knowledge.

An activity-practice component is treated as a cluster of scientific-research skills and competence used to solve the tasks within relevant knowledge and intellectual operations (synthesis, analysis, generalization, abstracting, caparisoning, concretizing etc). An activity-practice component integrates the following competences: methodological ones (application of the methods used to analyze socio-economic phenomena within the frame of the object studied; the processing of the experimental data generated on the basis of the mathematical methods, the quantitative and qualitative analyses of the empirical data; the interpretation of the results received; a the dynamic tracking of the aspect scrutinized); organizational work (the general plan compilation research, structuring logically the work to be done, the identification of the problem researched, its limits and decision framing, the target problem articulation); communicative ones (communication with a respondent, the correct application of mathematical notions both in oral and written speech, information searching both in printed and electronic versions).

A reflexive component includes many reflexive skills: self-awareness, comprehension, evaluation of both the process and the research activity results.

Personality-centered creative components, as we comprehend it, is the system of personal traits most crucial for researcher’s success (as well as for students’ creativity). We have defined the following features, which are significant in training SEA students: the ability to identify the problem and to solve it, to think originally, to draw their attention and rank it, to avoid a stereotype in decision-making, to study an issue from different points of view.

We have laid down the criteria for future SEA students to determine their potential of scientific-research work readiness. These criteria are conative (drive to success in a profession, enjoyment in work done, intrinsic motivation prevalence); conceptual-logical thinking (basic and complementary competence, deep insight and validity in arguments); operational (investigative behavior in a research task solution, an aptitude to use methods typical for the socio-economic study, to interpret the data received and to present them to an audience). self-evaluation (the ability to control their activities, to evaluate their competence, to apply contingency plan to meet the changing situations, critical appraisal of the methods used). subjective- creative (the ability to find, lay down and solve a problem, as well as to focus on and distribute their attention, independent thinking and the stereotype overcoming skills, original approaches in a problem solution, a turn for reviewing data in different ways).

Based on factors of preparedness for scientific research and criteria mentioned above, we have separated out and justified three levels of readiness, they are low, intermediate and high. The first-year students of Kursk state university, the faculty of philosophy, sociology and cultural science, (specialty “Sociology”) and the students of the faculty of economy and management (specialty “Human resource management”, “Organization management”, “Marketing”) took part in the survey conducted at the ascertaining stage of our experiment during 2015/2016 academic year. Eighty-one students took part in the sampling. Their primordial levels of readiness competences were determined through supervision, questionnaire survey, and semantic differential (cognitive) method as well as through rigidity of the adapted text versions and with help of the texts by Johnson and Mechrabian. As a result we have singled out the extent of constituent development and have offered summative assessment of the general students’ capabilities level in research work.

The ascertain results of the experiment show that only a small group (8.6%) demonstrated a high level of readiness, the majority gained an intermediate and a low level (46.9% and 44.5% proportionally). Only 13.6% of the respondents felt encouraged to scientific research work. Most art students (64.2%) do not find the possibility of theoretical mathematics knowledge realization in their future occupation. Three-fourths of the students (76.1%) are not able to answer questions asked in nonstandard way. Only one-fourths of them succeeds in expressing their view clearly and confidently.

The data received prove the advisability of goal-oriented activity to create an educational environment for SEA students, for them to succeed in generating skills in scientific research work in terms of higher education in mathematics.

Our team has elaborated the model of developing future SEA students’ readiness in research work through education in mathematics. (fig.1)

Drafting of the experiment conceptual stage implies an academic activity designed to form and develop crucial readiness components to fulfil scientific research. The story problems used in mathematical subjects learning allow mastering basic skill in mathematical simulation and in problem-heuristic situations.

The readiness model we have developed is implemented in three stages during three-term periods.

Figure 1
A formative and developing model of SEA students’ readiness for scientific research

The dialogical and collaborative principles are the main didactic concept at a reproductive-algorithmic stage.

The educational process includes different aspects to develop reflexive-communicative skills and oral speech in terms of mathematics. These aspects concern communication in the form of dialogues, i.e. the shared study of problem-heuristic situations in team, the task analyses with malicious mistakes in them, crosscheck-assignments with their appraisal according to the criteria available. An emphasis was accorded to the story problems with basic mathematical concepts in them and the way to use them in the context of their professions. The solution of similar tasks improves the skills and abilities related to analysis, comparison, main and subordinate data partitioning, and the presentations of an elementary practical situation in mathematical terms. It should be stressed, that the professional contexts intensify students’ cognitive activity in mathematical applications. One can treat it as a benchmark in self-directed work outside the classroom.

Partially productive stage makes a stress on the problem elements in didactic material handling. At the beginning of the course unit study, we used the introductory lectures with the problem-heuristic situations in them and the scientific-format lectures followed by the review sessions. The analyses of the mistakes done accompanied them as well. The focus was on dynamic tasks concerning professional context. These tasks bring up the issues to answer which the students must have definite abilities mandatory for their prospective research trades. These abilities are monitoring, generalizing and comparing, and hypothesis generating. Dynamic tasks are relevant to team and group work. Moreover, they provide a background for the learner-centered approach in the mathematical simulation context (Students’ individual work). An elective course «Mathematics in Expert Review” has been developed, the prime objective of which is to make students more familiar with mathematical methods in socioeconomics. Among other factors, we have added fuzzy logic elements and theory of sets, which are not included in the basic course in mathematics. The level of students’ involvement has dramatically improved and it has made it possible to engage students into research work outside classroom (workshops, student research society, scientific projects at the department).

The third one, experience-based and creative stage concerns further developed subjectness and self-directed activity, with creative personal qualities prevailing. At this stage students are engaged in developing creative research themes (the results are undefined), different situations demanding unconventional approach. The structure of lectures has some ‘willful’ mistakes in it, problem-heuristic situations, a bulk of research and dynamic tasks for self-directed learning (both individually and group form). The extracurricular research activity emphasized some creative dimensions, i.e. the students took part in the development of the teaching packages in mathematics, they carried out some independent research inside spontaneous groups and they reported at the scientific conference “Mathematics and its application in modern science and practice” the participants of which were students and post-graduate students.

The diagram given below correlates state-of-facts and formative assessments.(fig.2)

Figure 2
Comparative study of the dynamic in the students’ research readiness formation.

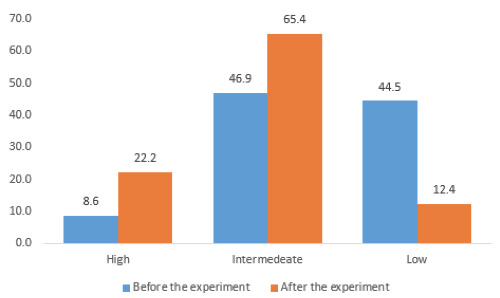
Results, when compared at both stages of the experiment, demonstrate noticeable improvement in the levels of research readiness: the number of the students with a low level has decreased by 3.1%. At the end of the experiment, most students (65.4%) confirmed their intermediate level of readiness. These changes have been analyzed based on Vilkokson’s T- criterion. The results of the educational experiment show positive changes in the readiness for scientific research work (confidence level is 0.95).

This progress results from some practicable judgments implemented into educational course and its coherence to the main educational experiment.

Moreover, through criterion we defined a noticeable discrepancy in the allocation of the research readiness components before and after the experiment. Total confidence in the results makes it possible to take them as a natural consequence of innovations used in first-year students’ training not as an incidental extension.

Thus, the data correlation of the summative vs formative results has proven this model and educational environment to be effective tools in training students’ research readiness.

4. Discussion

Based on quantity-quality data analysis delivered during educational experiment, we have found out some learning modalities, which intensify the efficiency of future SEA art students’ research readiness; they are axiological, organizational and reflexive-axiological issues.

4.1. Axiological issue

- The development of value perception of scientific-research work through exploring and mastering mathematical models of social phenomenon.

 Mathematical modeling allows the students to carry out research in theoretical area within their trades, to learn periodic and special literature, to pursuit creative search. The development of scientific-research awareness is a long, multi aspect and difficult process, with stable results through experience. Most Professors of Practice believe that the limits of scientific research work in higher education are within writing course and diploma papers. From our point of view this is a unilateral approach of training specialists. The efficiency of researchers’ awareness will increase if different relevant tasks with gradually added complication are included into the curricular for the first-year students. In particular, mastering the course of higher mathematics assume the employment of certain research-based and imitational tasks, which provide the description of different mathematical models in the context of future occupation. Educational process should drive students to understand the importance of SEA methods in their pursuit to be a responsive creative-oriented specialist, who is highly demanded in modern society.

- The creation of positive psychological atmosphere based on mutual respect and common creative activity in carrying out research and simulation-research tasks.

The subject of research is to be challenging and enjoyable. Success in work depends on the evidence of emotions involved. Recipathy and mutual respect are the most important factors in educational context. The teacher nowadays is to be skillful not only in interpreting scientific information but also in arranging advisees’ self-instructional work aimed at developing their skills and competence.

- The creation of a close collaboration and mutual support atmosphere through involvement of the students in common activity in classes as well as in self-instructional work.

SEA specialists are often involved in spitball analysis. The logicality of intellectual operation evolution is a triple-transcendence operation: from content to apprehension, then from apprehension to argumentation and, finally, from argumentation to function. Therefore, collective mental activity is reasonable enough in studying mathematics as it helps students to scrutinize variety of options, to argue for their positions, to cogitate at individual and group levels (i.e. to configure-to-levels, to state the compliance extent of the data gained to the aims of the research, to get the idea of their responsibility for team’s failure).

4.2. Organizational issues

 Gradual complication of the simulated research elements based on the mathematical models of the SEA phenomena, which prepare for the switch from traditional (reproductive) to innovative (problem-heuristic) way in didactic data handling.

Mathematical simulation offers challenging methods for SEA contemplation. The congruent methods and the models enclosed can be treated as a universal tool and they also pursuit the outcomes at an advanced level of formalization and characteristic generalization of the most important connections in studying economic phenomena and procedures. As a result, teaching student to apply mathematical simulation in social process analysis helps to prepare a better-trained specialist. The implementation of this approach in higher education has some advantages: it provides didactic component integration into different professional domains; it fosters positive motivation in research; it develops independence and autocriticism; it empowers students’ creative potentials. The professional-research situations should be gradually included into a curricular, with every succeeding model containing situations that are more complicated. In terms of an individual vector of scientific-research readiness formation one should move from practical dimension in reviewing mathematical concepts to independent task execution. A teacher is supposed to correlate the complexity level of text tasks and their control to individual students’ aptitudes. The process is to be of a didactic nature aimed at common creative work and realized in two main formats ‘teacher – student’ and ‘student – student’

According to A. Verbitsky, a dialogue rendering method intensifies the problematicity level and, as a result, stimulates the outcomes of education (Verbitsky 1960; Verbitsky 2004). Though problematicity is an indicative value of research work, we have found it feasible to study problem situations in both groups and the whole team. These tutorial methods promote research-thinking development, ability to communicate and intensify wide range of mental activity.

- The creation of interdisciplinary research environment combined with research work inside and outside classes.

In the context of a higher education, it is very important to form a team, the members in which share the same ideas, who are specialists in the same field and enjoy personal and professional communication within this team. A teacher should bolster students’ concern with research work during the term-time and during Off-Campus Employment to bring about some social-approval products (participation in conferences, material publishing and work at a project). A person who studies at educational institution (probably externally) must have a chance to estimate his or her merits in research, to reveal his or her creative potential and self-confidence in occupational ability. Therefore, it is quite reasonable to create interdisciplinary work-related research environment at academic institutions and integrate research both in class time and in extracurricular time.

- Outlook actualization within the depth and extent of aspects of mathematical knowledge in terms of practical work-related components.

The origin and development of research activities are stipulated by the need of an individual to get new information, to enhance obtained knowledge in definite subjects, to explore methods that are more effective in work. If through teacher’s appropriate endorsement a student realizes the way to define a forthcoming problem preceded by the analyses of task given by a teacher, student’s research readiness level will raise. The teacher is not believed to “digest” the material, but to deliver it in a rigorous thoughtful fashion to promote students to advanced level.

- Development and readiness level consideration, realization of complementary approaches – both individual and differential.

As most students at economic and sociological departments are interested in humanitarian subjects, it is reasonable to address their educational foundation and use con-centrum principle in-group work (i.e. material is assigned in relatively closed loop cycles).

4.3. Reflexive-axiological issues

- Image creation “I am a researcher” for future SEA specialists.

During investigation the authors of this paper encouraged students to evaluate their individual traits, their evolution habitude and adequacy in work-result precognition as well as their constant preprofessional self-realization in terms of their scientific readiness . Positive "I-concept” formation and its intensification perks up their confidence, nourishes their ability to realize their real and ideal “I-image”, treats adequately their current positions and prospects for future development.

- Development and enhancement of reflexive-research notions through research tasks realization, analysis of the available results as well as through the process of regulation and control of research behavior patterns.

A clearly articulated reflexive-research stand helps an individual communicate effectively with different group of people, adapt to a particular purpose or changing situation. A dialogue form is the most productive way to develop reflexive reasoning. It is very important to be able to check and control the process of work, to predict results, to adjust the strategy to research behavior patterns, to realize motivation of the other people and identify their principles in speculation. Negative-experience (errors and inaccuracy) analysis should be included in the educational process as this kind of activity is helpful in work apprehension and critical thinking. Moreover, a teacher is to inspire students for in-depth reflection, and avoid any formality in training no matter it concerns the students or the teacher. Internal discourse is the most advanced level. It can be gained on a parity-based discussion between a teacher and advisees, the former being active and encouraging students to be active as well.

– the monitoring of the level of their intellectual commitment, research creativity in different fields in terms of the work done.

5. Conclusion

Generation of scientific-research readiness in the course of mathematical sciences implies students’ performance of story problems, which contain mathematical model descriptions of socio-economic situations, research tasks, exercises with interdependent questions, creative tasks with the answers dependent on the judgement approach used. It is incompatible to use assessment criterion for any activity aimed at developing students’ creativity. The test, for example, focused on practicing research skills and awareness, may be only a free response test. Individuals with high creativity refer to research work as a source powerful intrinsic motivation: their mental intensity surpasses the use of intellectual endeavor, which is necessary to fulfil the task. A teacher must distinguish and support such kind of initiative, as is also a desire to solve a standard mathematical task in the original way and to track more efficient scenario. The activity in research of highly creative people has the form of intellectual initiative, and their mental activity is in progress beyond the pale necessary for problem solving. The process of story-problem solution should not reduce to a template, a ready-made algorithm, but it should suggest an innovative outlook and an unformulated version.

References

Belenchuk L. (2016). Historical pedagogical knowledge as a part of teaching culture. A teacher and professional becoming: historical experience of transfer of educational and cultural practice : International Research and Practice Conference materials - XXXI session of Academic Council for the problems in history of education and pedagogics at the Educational Academy of Russia. Tver: Otrok, pp. 56-62.

Korzhuyev, A. (2009). Modern theory of education: general scientific interpretation: manual for higher educational and post-graduate teachers’ studies institutions. Moscow: Academichesky prospect, pp. 192.

Nikitina, E. (2014a). Social-oriented programs in Polythechnical Museum practice. Scientific-theoretical and applied aspects in museum pedagogics: monographic edited volume. Moscow: Publishing house of the Institute of Effective Technologies, pp. 197-201.

Nikitina, E. (2014b). Project and T&E activity at school 1466 named after Yu. Rusheva. Science- and-theoretical applied aspects in museum pedagogics: monograph digest. Moscow: Publishing house of the Institute of Effective Technogies, pp. 288-300.

Nikitina, E. (2016) A. M. Novikov and the problems of vocational education in Russia. (historical and pedagogical context). Methodology of vocational education. International Research and Practice Conference materials. Moscow: Institute of strategies in education development RAE, pp. 121-125.

Prosolupova, N. (2011). Self-directed learning mathematics as a mean to form scientific research readiness of future specialists in socio-economics. Bulletin of VSU series of papers “Higher Education Issues”, 1, 38-40

Sergeeva M. (2015). Competency-based model of a graduator in terms of continuous vocational training: Monograph. Moscow: NOU HPE MIL, pp. 180.

Sukhodimtseva, A. (2014). Network projects as an innovative form of lifelong education. Lifelong le learning: Continuous education for sustainable development Proceedings of the 12th International Conference: in 2 parts. St. Petersburg: Pushkin Leningrad State University, pp. 73-75

Sukhodimtseva, A. (2016). The problem of metadisciplinarity in education in context of knowledge domain retention. International Research and Practice Conference materials “Phycology and pedagogics in educational and scientific aspects”, 17 September 2017, Surgut. Sterlitamak: International research agency, pp. 190

Sukhodimtseva, A. (2017). Educational methods updating in frames of educational training methodology. Teaching history and social sciences at school, 6, 39-43.

Verbitsky, A. (1960). A person in speech context: forms and methods of active learning. Moscow: Znaniye, pp. 63.

Verbitsky, A. (2004). Competency-based approach and context teaching theory. Moscow: Research Center of the Quality Problems in Training Specialists, pp.84.

1. Peoples' Friendship University of Russia (RUDN University), 117198, Russia, Moscow, Miklukho-Maklaya st., 6; E-mail: sergeeva198262@mail.ru

2. Peoples' Friendship University of Russia (RUDN University), 117198, Russia, Moscow, Miklukho-Maklaya st., 6

3. Peoples' Friendship University of Russia (RUDN University), 117198, Russia, Moscow, Miklukho-Maklaya st., 6

4. Peoples' Friendship University of Russia (RUDN University), 117198, Russia, Moscow, Miklukho-Maklaya st., 6

5. Peoples' Friendship University of Russia (RUDN University), 117198, Russia, Moscow, Miklukho-Maklaya st., 6

6. Peoples' Friendship University of Russia (RUDN University), 117198, Russia, Moscow, Miklukho-Maklaya st., 6